Normal Approximations

STAT 20: Introduction to Probability and Statistics

Concept Questions

01:00

A die will be rolled \(n\) times and the object is to guess the total number of spots in \(n\) rolls, and you choose \(n\) to be either 50 or 100. There is a one-dollar penalty for each spot that the guess is off. For instance, if you guess 200, and the total is 215, then you lose 15 dollars. Which do you prefer? 50 throws, or 100?*

Which do you prefer? \(n = 50\) rolls, or \(n = 100\) rolls?

* From the text Statistics by Freedman, Pisani, and Purves

Prefer 50 because the square root law tells us the the spread of the distribution will be smaller for fewer rolls. If we were computing the average number of spots, then we would pick larger \(n\) since \(\sqrt{n}\) goes in the denominator of SE.

02:00

One hundred draws will be made with replacement from a box with tickets \(\fbox{0}\, \fbox{2}\, \fbox{3} \,\fbox{4} \,\fbox{6}\). Which of the following statements are true? *

• The expected value of the sum of the one hundred draws is 300, give or take 20 or so.
• The expected value of the sum of the one hundred is 300.
• The sum of the one hundred draws is 300, give or take 20 or so.
• The sum of the one hundred draws is 300.

* From the text Statistics by Freedman, Pisani, and Purves

The EV is a constant, and note that the box average is (0+2+3+4+6)/5 = 3, so the EV of a single draw is 3, and the EV of the sum of 100 draws is \(100\times 4 = 300\). The give or take number given here is the box SD \(\times 10 = 2\times 10 = 20\). You can let \(X\) be a random variable that is the value of one ticket drawn at random from this box, and then we want \(S_{100}\). This can be boardwork.

01:00

We have two random variables: \(X \sim\) Binomial(\(10, 0.2\)) and \(Y\) is the random variable that is the value of one ticket drawn at random from a box with tickets \(\fbox{0}\, \fbox{2}\, \fbox{3} \,\fbox{4} \,\fbox{6}\).

We take the sum of 100 iid random variables for each of \(X\) and \(Y\), called \(SX_{100}\) and \(SY_{100}\). The empirical distributions of \(SX_{100}\) and \(SY_{100}\) are plotted below.

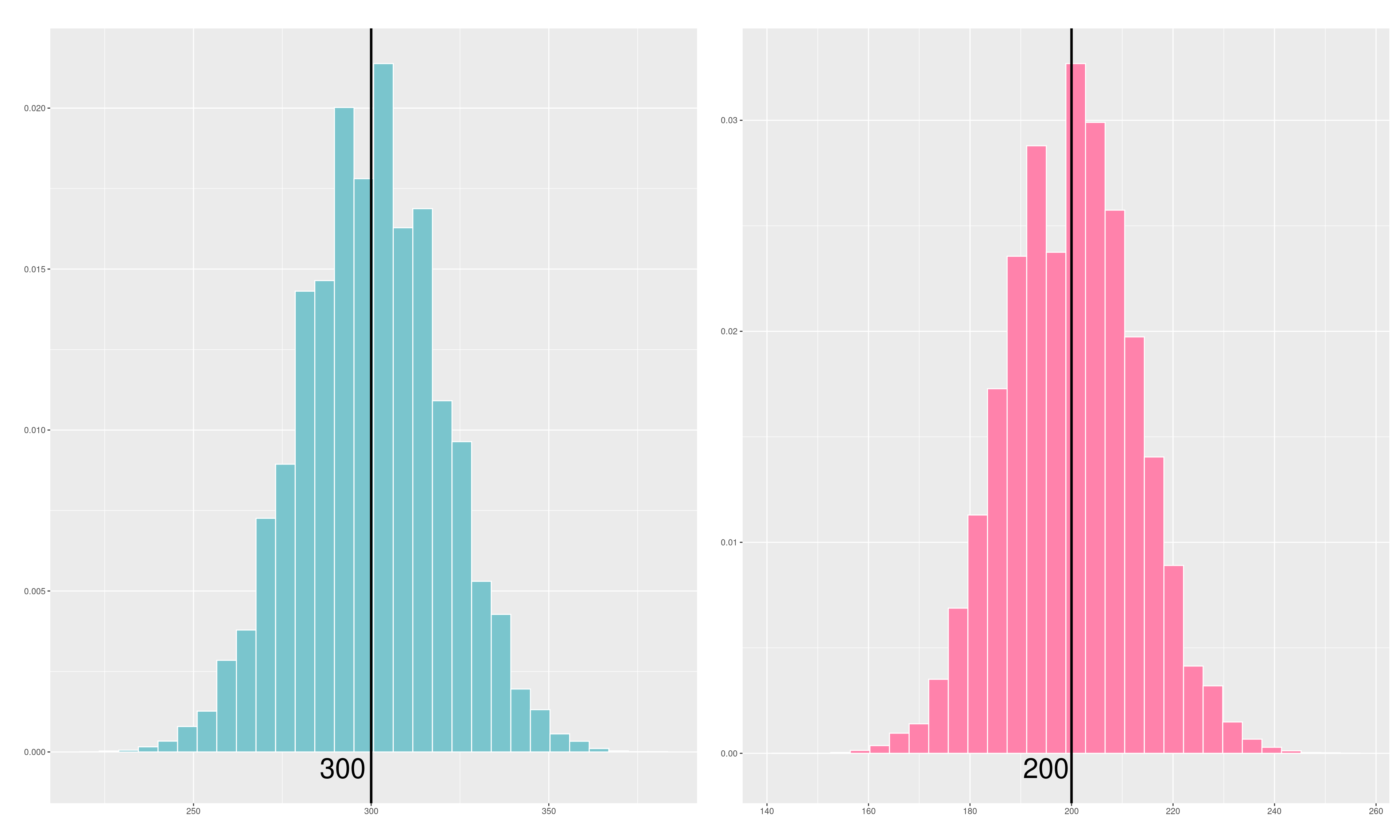
Which distribution belongs to which random variable?

They need to remember that the expected value of S_x will be 200, and for S_y 300.

Quiz review

25:00

Break

05:00